Conic Sections

What are they?

First off, what a weird name! Conic Sections? Why do they call them that? The formulas on this page are called "conic sections" because they represent shapes that result from slicing a cone and observing the resulting sections. Does that make sense? Imagine you are looking at a traffic cone. If you also happen to be holding a small chain saw (and really why wouldn't you be?) and you fire it up and slice off the top half of the cone straight across, then the rims of the resulting cross sections will be in the shape of—that's right!—circles. A circle is therefore a "conic section", because it derives from those sections of a cone. If you perform a similar slice across the top of the cone, but this time you behead it at an angle, the rims of the resulting cross sections will be elongated circles called—right again!—ellipses. Are you picturing yourself with the chain saw, producing these shapes? If so, how will you make a parabola? Easy! Just follow proper operating specs with the blade perfectly vertical and slice off the front, side, or back of the cone, as if you are cutting a little door for an inner cave. What shape will the rims of the resulting cross sections of the cone be now? Parabolas! What about hyperbolas? All they really are is circles or ellipses that have been sliced perfectly in half and then the halves are reversed to face away from each other. Can you figure out how to carve up a traffic cone for that result? If so, you have a promising career ahead as a lumberjack, or menace to society. I welcome your replies about how to do it. I also invite you to click the image in this paragraph to check out my book of spiritual writings, featuring—of all things—traffic cones.

Standard Format Equations

Parabolas:

The standard format for the equation of a parabola is:

Circles:

The standard format for the equation of a circle is:

Ellipses:

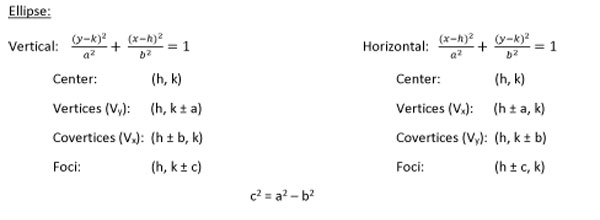
The standard format for the equation of an ellipse is:

Hyperbolas:

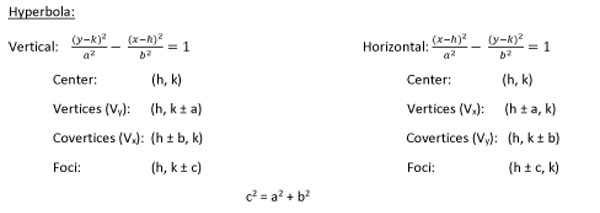
The standard format for the equation of a hyperbola is:

Shape Identification

Determining Shapes From Equations:

For equations in the following format, you can use details about the coefficients of the most powerful terms to quickly identify graph shape: